Mathematics | Agency of Education

In 2010, Vermont adopted the Common Core State Standards for Mathematics. The CCSS for Mathematics provide clear, consistent expectations for students, which define what students should understand and be able to do. The Agency of Education has developed a Proficiency-Based Graduation Requirement Hierarchy for mathematics based on the CCSS.

Summer 2025 Grant Opportunity

The AOE is dispersing grant funds in order to support SUs/SDs in providing an opportunity to complement and/or amplify their existing middle school and high school professional learning needs through an opportunity for summer professional learning. Please click on the following link to learn more about this upcoming grant, including timelines for submission.

Professional Learning for Secondary Math Educators Grant Overview

Section List

Spotlight on Equity Resources

The Portrait of a Graduate as a Function of Math

Mathematics Proficiency-Based Learning Hierarchy

Vermont-Specific Professional Learning Resources

Standards

Performance Assessments

Resources

Newsletters

Professional Organizations

Spotlight on Equity Resources

Educational equity means that every student has access to the resources, opportunities, and educational rigor they need at the right moment in their education, whatever their race, gender/identity, sexual orientation, ethnicity, religion, language, ability, family background, or family income may be. (Adapted from CCSSO, Leading for Equity.) The Mathematics: Spotlight on Equity Resources provides a list of considerations and resources for the purpose of supporting equity and access while emphasizing high-quality and culturally sustaining learning opportunities for all students.

Return to Section List

The Portrait of a Graduate as a Function of Math

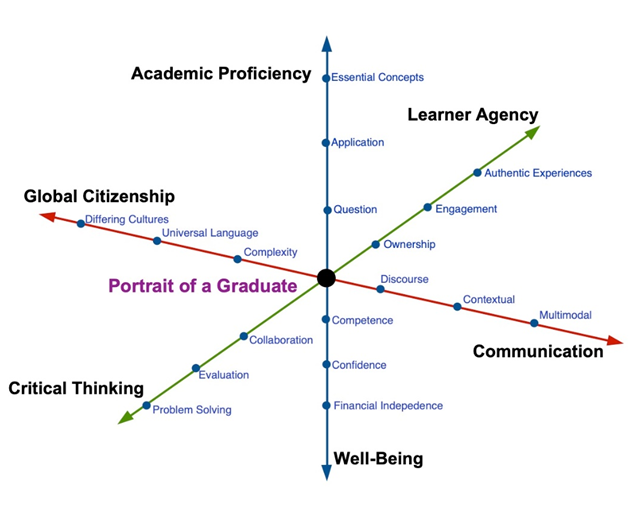
A Vermont Portrait of a Graduate (PoG) includes six attributes: learner agency, global citizenship, academic proficiency, communication, critical thinking, and well-being. Students can gain experience towards proficiency in all of these areas during their study of mathematics. (A Vermont Portrait of a Graduate as a Function of Math)

The diagram below depicts how a student in the study of mathematics will address the six attributes described in the Vermont PoG. The information contained in the diagram was largely inspired by the performance indicators of the Vermont PoG’s attributes and also from the Equitable Mathematics Teaching Practices found in the book Catalyzing Change published by the National Council of Teachers of Mathematics.

The Portrait of a Graduate as a Function of Math

Return to Section List

Mathematics Proficiency-Based Learning Hierarchy

Grades 9-12 Mathematics Proficiency-Based Graduation Requirement Hierarchy

The Proficiency-Based Graduation Learning Hierarchies support equity by providing a cohesive and coordinated vision of student-centered learning across Vermont schools. The hierarchies serve as a foundation for the implementation of standards adopted by the Vermont State Board of Education, Local Comprehensive Assessment Systems, flexible pathways, and personalized learning plans. While the Priority Performance Indicators list only content standard clusters, the mathematics practice standards outlined in the CCSS-M are equally important. The practice standards are:  

• Make sense of problems and persevere in solving them.  

• Reason abstractly and quantitatively.  

• Construct viable arguments and critique the reasoning of others.  

• Model with mathematics, Use appropriate tools strategically. 

 • Attend to precision.  

• Look for and make use of structure.  

• Look for and express regularity in repeated reasoning.  

A mathematically literate person is proficient in the areas outlined in the content-based PPIs and is also skilled in the habits and ways of working outlined in the practice standards. Many of the practice standards also connect easily to the Transferable Skills Graduation Requirements. 

Grades K-8

The K-8 math grade-level hierarchies are designed to build a strong foundation for students to meet the Proficiency-Based Graduation Requirements (PBGR) in mathematics. These hierarchies focus on four Critical Proficiencies: Quantitative Reasoning, Algebraic Reasoning, Geometric Reasoning, and Measurement and Data, with Statistical Reasoning emphasized in grades 6-8. Critical Proficiencies are directly aligned to the PBGR Critical Proficiencies for high school and largely follow the structure of domains from the Common Core State Standards-Mathematics (CCSS-M). This structured progression ensures that students develop the essential skills needed for success in high school and beyond. The prioritization draws heavily from the SAP Achieve the Core Focus by Grade Level content delineated as Major Work and also reflects direct input from educators in the field, ensuring relevance and effectiveness in meeting students' learning needs.  

Some content identified as Supporting or Additional was included each grade level within Geometric Reasoning, as these standards become Major Work by eighth grade and beyond. Selected standards related to Measurement and Data (and Statistics and Probability in grades 6-8) were also included to better prepare students for high school, college, and career readiness. Reports such as the PISA (2022) highlight the need for increased data literacy1, related to math literacy in the real world. Also considered were the High Leverage Concepts according to the All Learners Network (ALN). Finally, and most importantly, input from over 15 SU/SDs was gathered through polling to identify priority cluster choices for each grade level. This feedback, along with insights from multiple review sessions with educators, was carefully considered. 

While the following grade-level hierarchies represent the content that should be emphasized and prioritized, all other content described by the CCSS-M at each grade level should still be addressed to build comprehensive math literacy. Through the internal and field review process, several revisions were made to the SAP Achieve the Core Focus by Grade Level content framework and are summarized on each grade-level hierarchy.

The Agency of Education (AOE) recognizes the considerable time and effort that educators and other stakeholders across the state have already put forth developing proficiency-based learning systems and this work is intended to serve as a resource for SU/SDs to consider as they reflect on the key concepts and skills that students should develop within each content area. The tool, Priority Performance Indicators Quality Criteria  | Agency of Education, can be used to help evaluate Priority Performance Indicators.

Vermont-Specific Professional Learning Resources

Essential Math for College and Careers

Essential Math for College and Careers (EMC2) is an innovative math course designed for high school seniors who have completed Algebra I, Geometry, Algebra II, or the equivalent but still need to strengthen their math preparedness to be ready to launch into technical training, college, or career. 

The course was developed by Vermont Student Assistance Corporation (VSAC) GEAR UP, the Vermont Agency of Education (AOE), and the Vermont State College System (VSCS), together with high school and postsecondary math faculty, and is designed to be project-based with guided student discussion. Concepts are not taught by giving students algorithms but rather by discovering those algorithms within a set of rich problem-based tasks.

EMC2 is currently taught at four Vermont high schools as well as through the Vermont Virtual Learning Collaborative (EMC2 through VTVLC is free of charge through the 24-25 school year). EMC2 is recruiting additional schools for the 2024-2025 school year. 

For more information, or to find out how your school can participate, visit the EMC2 website.

Free Professional Learning from All Learners Network

All Learners Network (ALN), with the support of the AOE provides a wide variety of workshops and an annual conference all centered around high-quality professional learning about teaching mathematics. ALN’s events can be found on All Learners Network website and any of the events that list VT AOE in the title are free of charge to all Vermont educators.

Return to Section List

Professional Organizations

NCSM: National Council of Supervisors of Mathematics
NCTM: National Council of Teachers of Mathematics
VCTM: Vermont Council of Teachers of Mathematics

Return to Section List

Questions?

Email aoe.pblhelpdesk@vermont.gov